Consecutive first step reversible reactions

A turns into B, with rate constant k₁, which turns into C, with rate constant k₂. Now, B can turn back into A, with rate constant k_-1.

The problem of integrating the rate equations can be overcome by the use of approximations (P. W. Atkins, Physical Chemistry, Oxford University Press). An important approach is the Steady-State Approximation that assumes that the concentration of B does not alter. This simplification leads to analytical solutions to the rate equations.

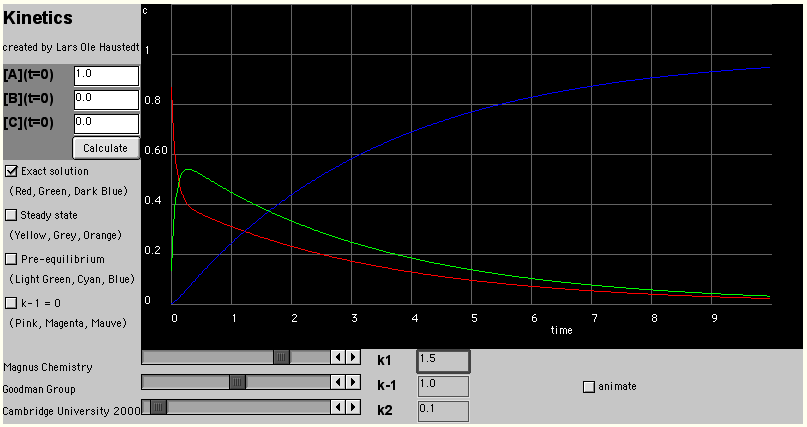
The approximation will be best if the concentration of B stays approximately constant, which means that the sum of k_-1 and k₂ must be greater than k₁ if the initial concentration of B is zero, and it must be equal to k₁ if the initial concentration is not zero. This seems a stringent test. The applet makes the comparison in a visual way. The figure shows the changes in concentration of A, B, and C as the reaction proceeds, with k₁ = 1.5, k_-1 = 1.0, and k₂ = 0.1. This is a very bad case for the steady state approximation, as the rate constant for the formation of B is greater than the rate constants for its destruction. As a result, the concentration of B builds up as the reaction proceeds, before eventually draining into C. The steady state approximation assumes that the concentration of B does not change, and so it stays at its initial concentration of zero. This gives a very different reaction profile. Experimentation with the applet shows that the approximation works well if k₁ is smaller than k_-1 and k₂ as expected. How much smaller do they need to be? Can one be larger and the other smaller? The answer can be found by adjusting the settings of the applet.

An alternative approach to solving the rate equations is to use the Pre-equilibrium Approximation. This is that A and B are always present in their equilibrium ratios, which is equivalent to assuming that k₂ is much slower than both k₁ and k_-1.

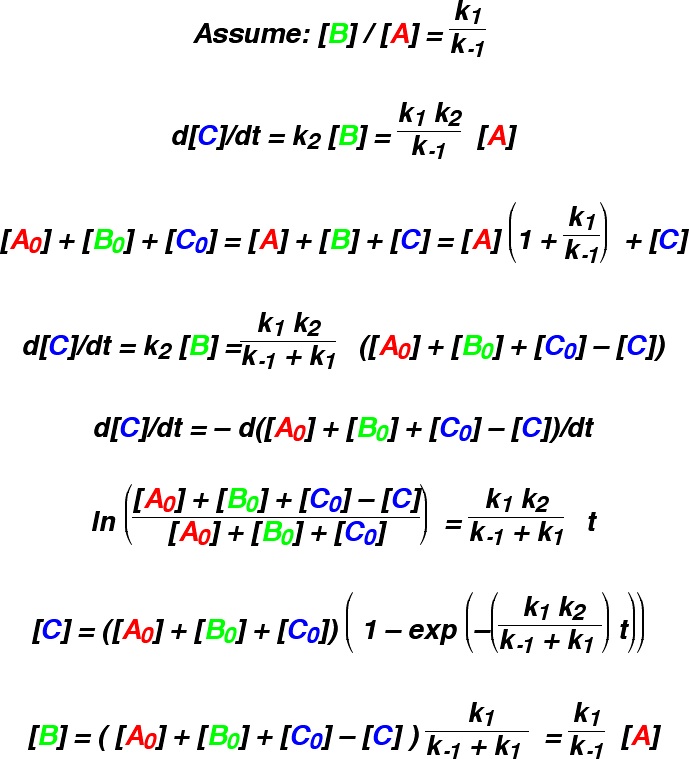
The applet also draws the results of making this assumption. A and B immediately change from their initial concentrations to the equilibrium concentrations, and then both gradually diminish as C forms. This works best if k₁ and k_-1 are fast, and the applet helps to answer the question: how fast? The approximation is poor at the beginning of the reaction, as A and B reach equilibrium too fast. However, it often traces the rest of the reaction quite well.

Neither of these approximations reproduces the gradual increase and decrease in the concentration of B that occurs for may choices of k₁, k_-1 and k₂. Another approximation is to assume that k-1 is zero. This may appear a drastic approximation, but it leads to rate equations which can be solved analytically.

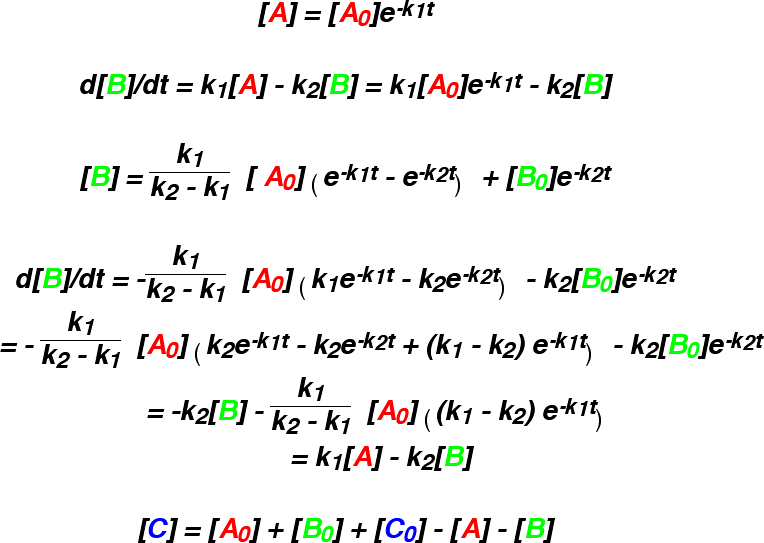
This approximation gives curves which are of the same general form as the numeric results. If k_-1 is zero, then the numeric and analytical solutions coincide. However, if k_-1 is not zero, B forms too quickly.

The same applet may also be used to investigate thermodynamic and kinetic control. If the initial conditions are set so that A and C have a concentration of zero, and B has a concentration of one, then the equations correspond to a common situation where a starting material may react to form a kinetically favoured product, or a different thermodynamically favoured product. How long will it take to get the maximum yield of the kinetic product? At what point will the thermodynamic product become the main component of the mixture? These questions can be answered by adjusting the parameters of the applet

This applet allows students to explore the effect of various kinetic regimes, by displaying the solutions of rate equations, solved by numerical means, and comparing them with the results of various approximations.